Role of the Coulomb Potential in Compton Scattering
|
We report a fully differential study of ionization of the Ne L shell by Compton scattering of 20 keV photons. We find two physical mechanisms that modify the Compton-electron emission. Firstly, we observe scattering of the Compton electrons at their parent nucleus. Secondly, we find a distinct maximum in the electron momentum distribution close-to-zero momentum that we attribute to a focusing of the electrons by the Coulomb potential.
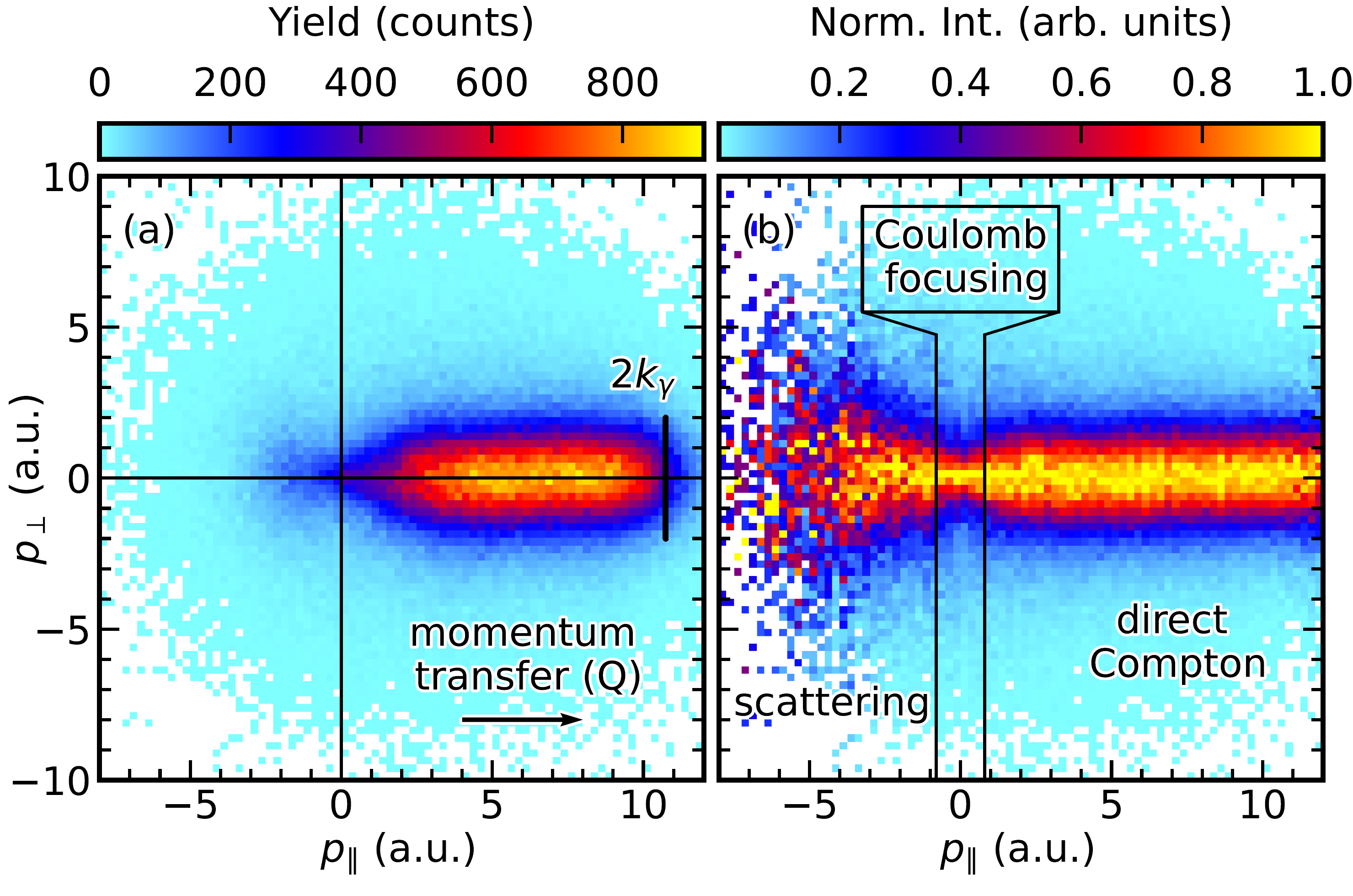
Figure 1: Momentum distribution of Compton electrons recorded at E𝛾 = 20 keV (k𝛾 = 5.37 a.u.) for the Ne 2s and 2p shells. Horizontal axis: electron momentum parallel to the momentum transfer Q (∥). Vertical axis: electron momentum in one direction perpendicular to the momentum transfer Q (⊥). The data are integrated over the third electron momentum component and all photon momentum transfers. (a) The momentum distribution. The maximum momentum transfer (i.e., photon backscattering) is approximately 2k𝛾, as indicated in the panel. (b) Same as panel (a) but each column of the histogram is normalized to its maximum. Three electron momentum regions dominated by different mechanisms are indicated: Direct Compton, Coulomb focusing and Scattering.
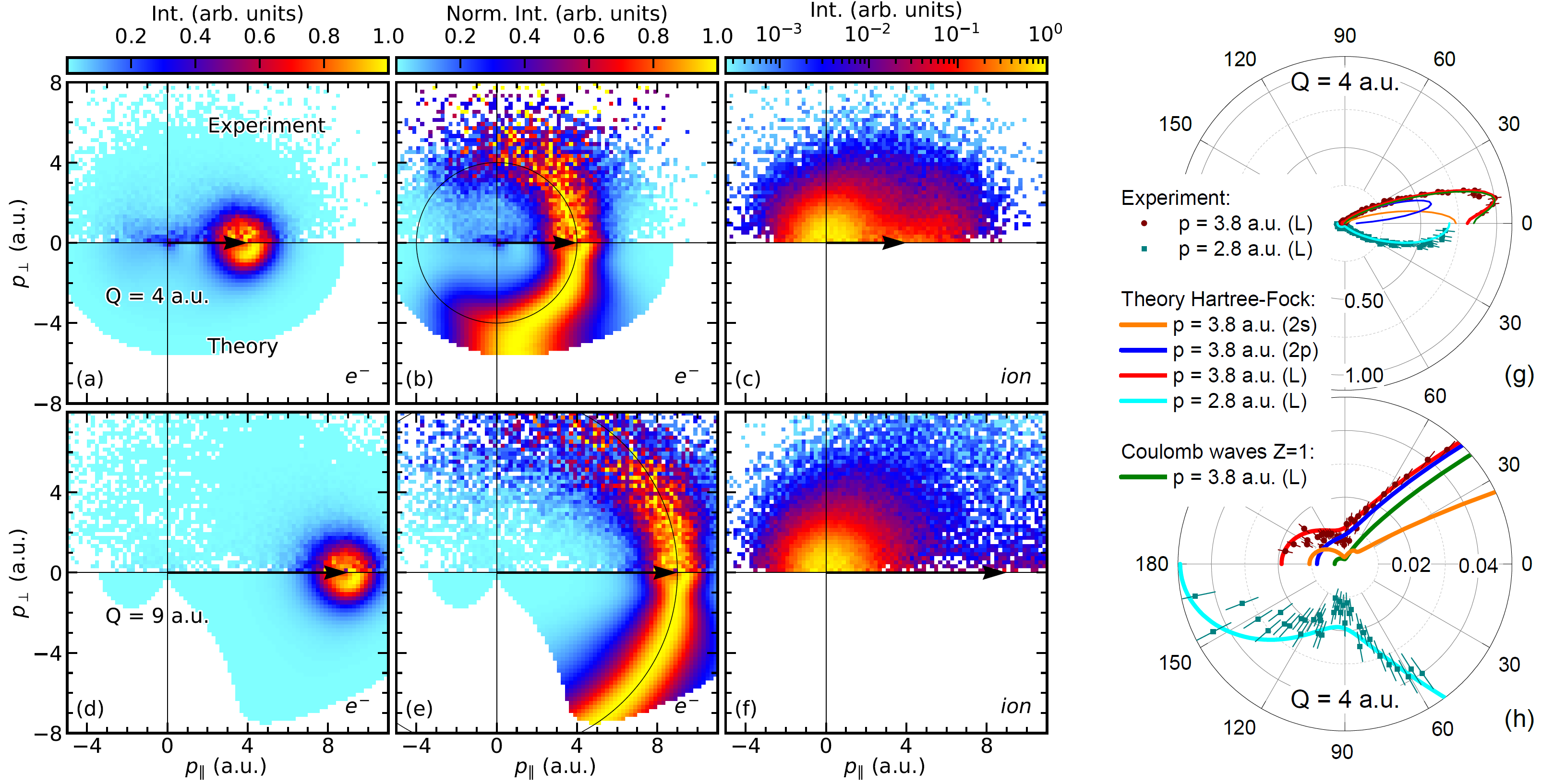
Figure 2: Momentum distribution of Compton electrons (a),(b),(d),(e) and ions (c),(f) recorded at E𝛾 = 20 keV (k𝛾 = 5.37 a.u.) for the Ne 2s and 2p shells for fixed momentum transfer of 3.5 a.u. < Q < 4.5 a.u. (a)–(c) and 8.5 a.u. < Q < 9.5 a.u. (d)–(f), as indicated by the black arrows. Horizontal axis: electron (ion) momentum parallel to the momentum transfer (∥). Vertical axis: electron (ion) momentum perpendicular to the momentum transfer (⊥). The upper half of panels (a),(b),(d),(e) show the experimental data, the lower half the theoretical results evaluating Eq. (1) in the A2 approximation. (a),(c),(d),(f) The color coding shows the differential cross section dσ(p∥,p⊥)/dp∥dp⊥ × 1/p⊥. (b),(e) The same as panels (a),(d) but each row is normalized to its maximum value. The circles indicate the locus of events when the electron with the given momentum transfer is elastically scattered at the nucleus. (c),(f) Counts are shown on a logarithmic scale. (g),(h) Polar representation of the normalized emission probability distributions, obtained for photon momentum transfers 3.5 a.u. < Q < 4.5 a.u. and electron momenta p = 3.8 a.u. and 2.8 a.u. The experiment shows the results for the L shell, the computed distributions are given separately for 2s, 2p electrons, and the combined L shell. Results from Hartree-Fock calculations for Ne+, as well as from modeling the continuum with Coulomb waves are depicted (see legend). All error bars indicate the standard statistical error. (g) Full distributions. (h) Distributions of panel (g) but enlarged by a factor of 20 around the origin.
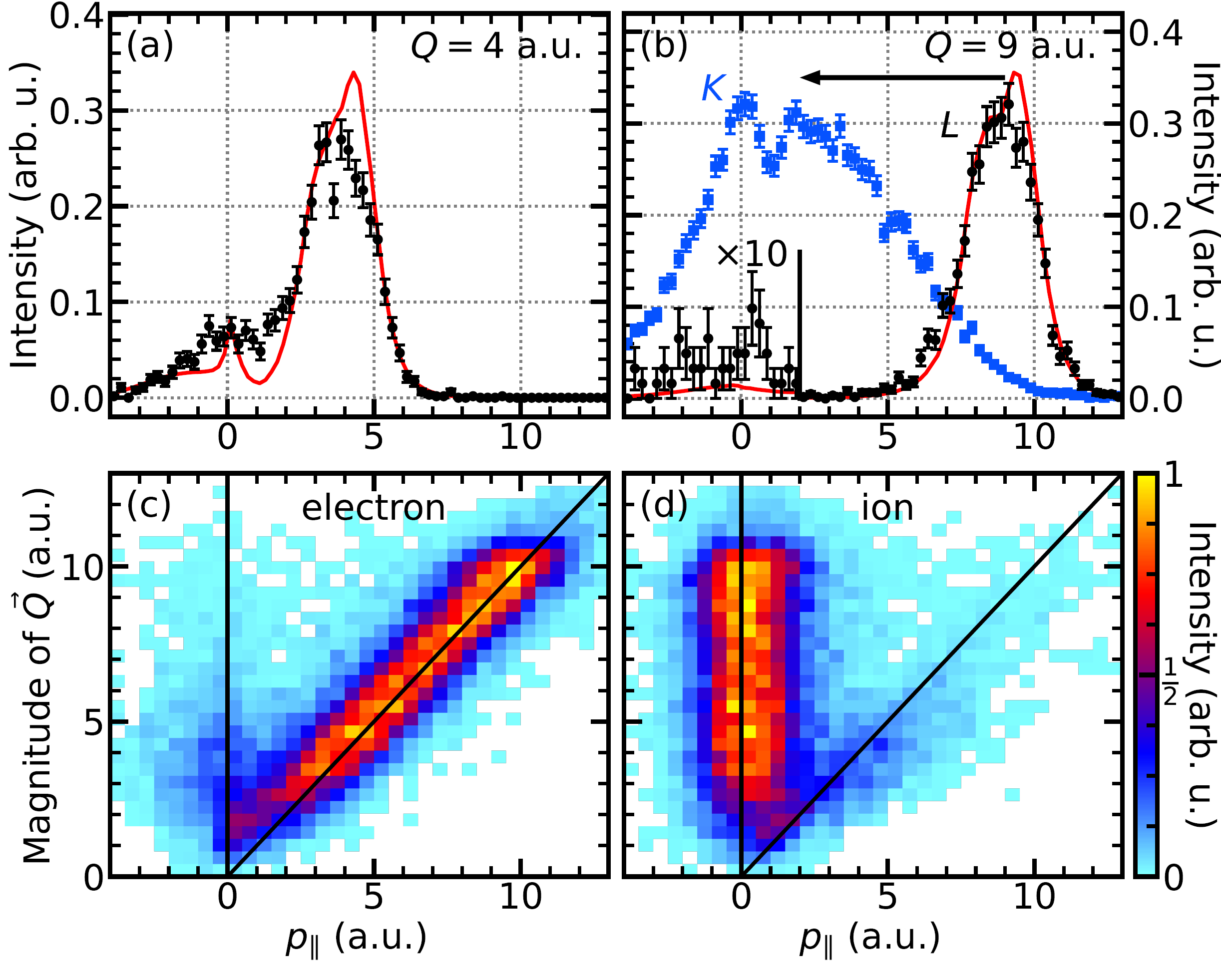
Figure 3: Electron and ion momentum balance parallel to the momentum transfer for Compton scattering recorded at E𝛾 = 20 keV (k𝛾 = 5.37 a.u.) for the Ne 1s [panel (b) only], 2s, and 2s shells. Horizontal axis: momentum parallel to the momentum transfer (∥). Vertical axis: intensity in panels (a),(b), Q in panels (c),(d). Electron data are shown in panels (a),(b),(c), ion data in panel (d). Panel (a) shows momentum transfers where 3.5 a.u. < Q < 4.5 a.u., panel (b) shows 8.5 a.u. < Q < 9.5 a.u. (a),(b) Black points show 2s/2p-electron data; red lines corresponding theory calculations. All error bars show the standard statistical error. (b) Blue points show Ne 1s-electron data. For small p∥ the 2s/2p-data is scaled by a factor of 10. (c),(d) Vertical and diagonal lines indicate different mechanisms. The data is restricted to a momentum component perpendicular to 𝑄 of |p⊥| < 0.4 a.u.
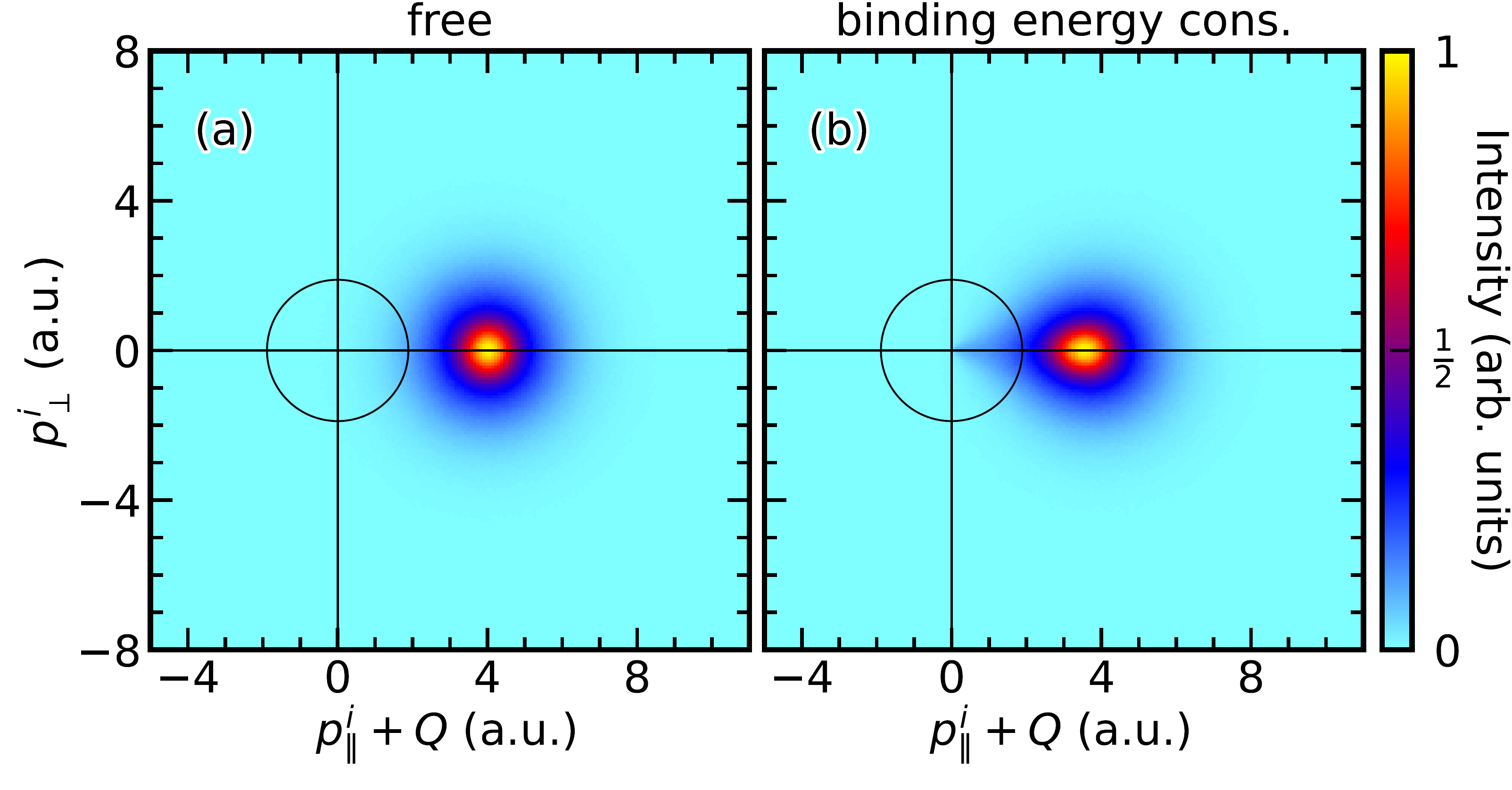
Figure 4: Classical modeling of the Coulomb focusing. (a) Momentum distribution of Ne 2s and 2p shells shifted by a momentum transfer Q = 4 a.u. The circle has a radius of √2Ip, thus only electrons outside this circle can overcome the binding energy. (b) For each electron in panel (a), the kinetic energy is reduced by the binding energy, while keeping the initial direction of the momentum vector. Electrons within the circle in panel (a) have, thus, a negative energy and are not shown in panel (b). Electrons on the surface of the sphere in panel (a) are focused to the origin in panel (b).
Publication: |